ベクトル解析

最終更新日：2024.3.26 (旧版 2023.4.26)

物理現象を記述するのにベクトル解析が多用されます。でも、ベクトルは苦手だし、それの微分・積分となるとお手上げの人が多いかと。ここでは、主なベクトル微分演算子をリストしてみました。
Vector analysis is often used to describe physical phenomena. However, many of us are not very good at vectors, and when it comes to the derivatives and integrals of vectors, many of us are not very good at it. Here is a list of the main vector differential operators.

---

ベクトル解析：ベクトル微分演算子

---

グラジエント(gradient)：勾配

グラジエントはスカラー量に作用するベクトル微分演算子です。演算後の量はベクトル量です。𝑓の作用する関数の変化(傾き)が最大となる方向を表ます。
The gradient is a vector differential operator acting on a scalar quantity. The quantity after the operation is a vector quantity; it represents the direction in which the change (slope) of the function on which 𝑓 acts is maximal.

---

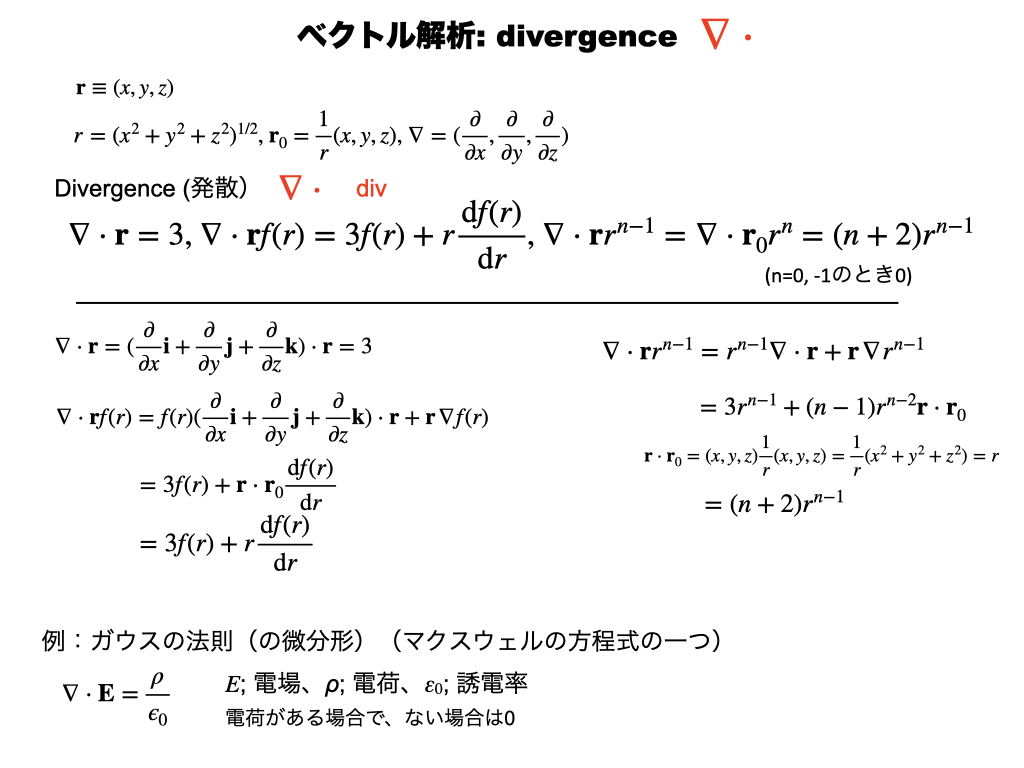
ダイバージェンス(divergence)：発散

ダイバージェンスはベクトル量に作用するベクトル微分演算子です。演算後の量はスカラー量になります。作用する関数の「湧き出し」，「増分」，「収支バランス」を表ます。
Divergence is a vector differential operator that acts on vector quantities. The quantity after the operation is a scalar quantity. It represents the "outflow," "increment," or "balance" of the function it acts on.

---

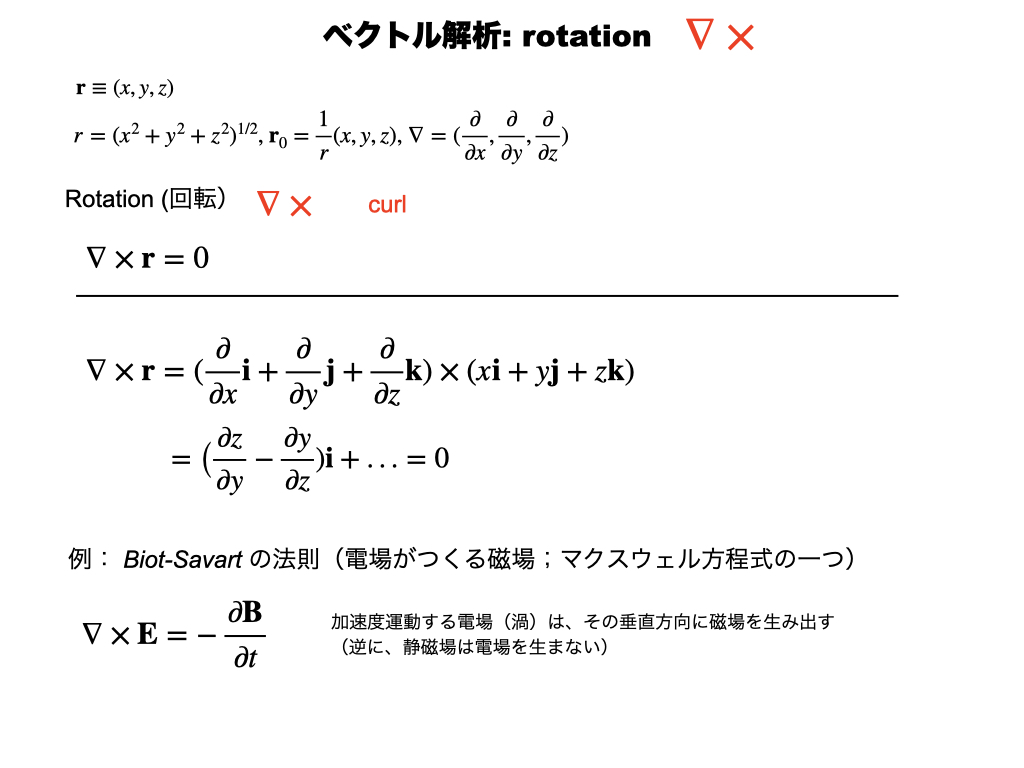
ローテーション(rotation)、カール(curl)：回転

ローテーションはベクトル量に作用するベクトル微分演算子です。演算後の量は　ベクトル量になります。作用する関数の「渦（うず）」を表ます。
Rotation is a vector differential operator that acts on a vector quantity. The resulting quantity is a vector quantity. It represents the "vortex" of the function it acts on.

---

ベクトル場の２階微分

ベクトル場の微分には、いくつかの有意な２階微分があります。それを列記しましょう。
ファインマン物理学　III　電磁気学　岩波書店　宮島　龍興 訳　より
There are several significant second-order derivatives of vector fields. Let us list them.

---

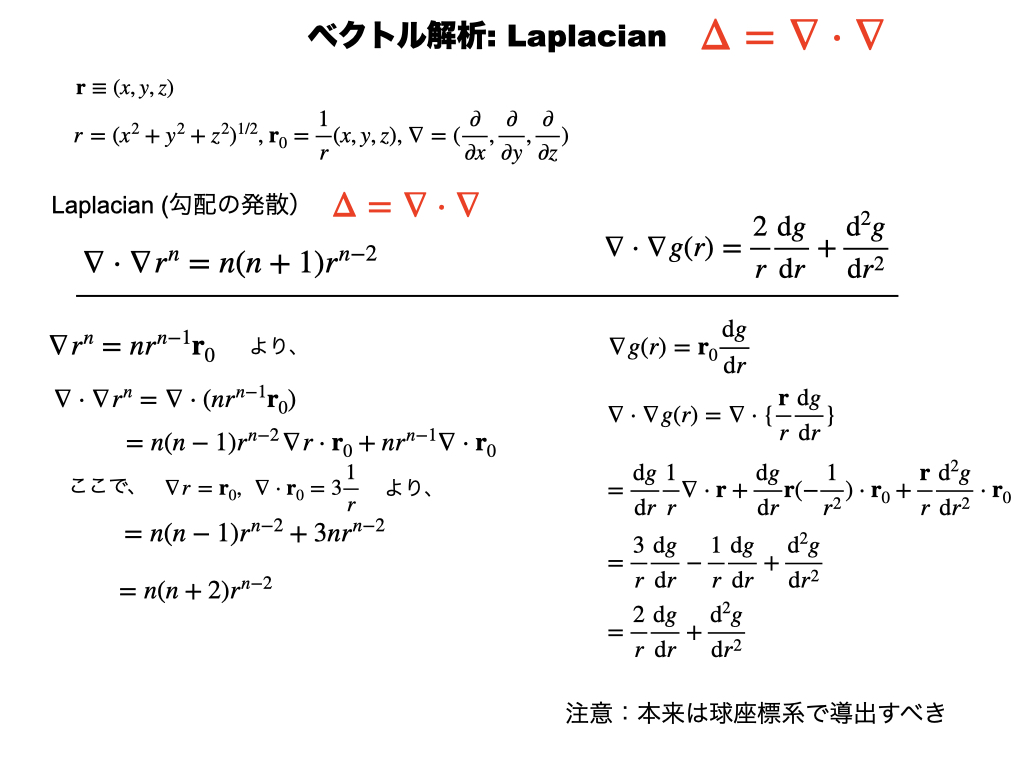
ラプラシアン(Laplacian)

ベクトル場の２階微分でよく使われるのがラプラシアンです。スカラー量に作用し、演算後の量はスカラー量で、作用する関数の「へこみ」，「たわみ」を表ます。
The Laplacian is often used in the second-order derivative of vector fields. It acts on a scalar quantity, and the resulting quantity is a scalar quantity that represents the "dent" or "deflection" of the function it acts on.

---