非粒子系からの散乱 (scattering from nonparticular systems)

最終更新日：2023.5.2

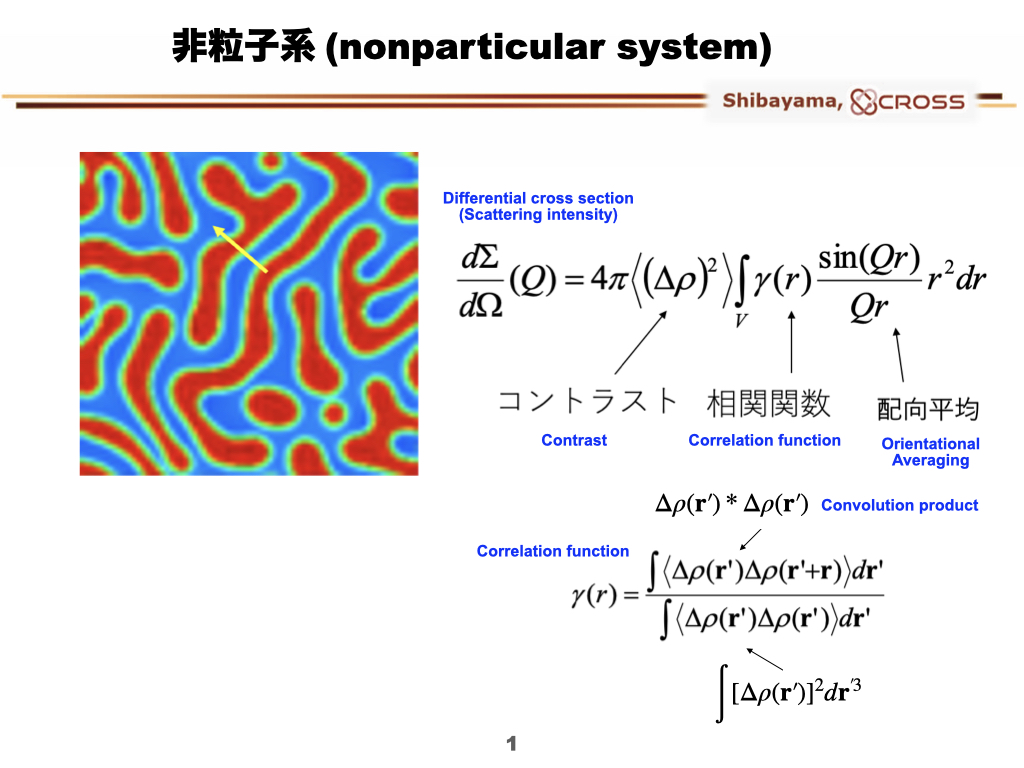
球状粒子や棒状粒子からの散乱のように散乱体の形状がわかっている場合は、その物体のフーリエ変換を行うことで散乱関数が計算できますが、形状が未定の場合や、特定の形を持たない２相系の場合では、統計的に散乱関数を求めることになります。この統計量に相当するのが相関関数 γ(r)です。図は不定形の２相系の模式図を示しています。このような場合、散乱長密度差 Δρ(r)の自己畳み込みにより相関関数 γ(r)が定義されます。そして、そのフーリエ変換により散乱強度関数が求まります。豹柄や虎柄の相関関数や散乱強度を求めてみたら面白いですね。
If the shape of the scatterer is known, as in the case of scattering from spherical or rod-shaped particles, the scattering function can be calculated by performing the Fourier transform of the object. However, if the shape is undetermined or for a two-phase system without a specific shape, the scattering function is obtained statistically. Correlation function γ(r) corresponds to this statistic. The figure shows a schematic of a two-phase system with an indefinite shape. In such a case, the correlation function γ(r) is defined by the self convolution of the scattering length density difference Δρ(r). Then, its Fourier transform yields the scattering intensity function. It would be interesting to find the correlation function and scattering intensity for leopard or tiger patterns.

---

---